Downtown Helsinki I stepped on a pedestrian street tiled with the standard nonperiodic Penrose (kite and dart) tiling.
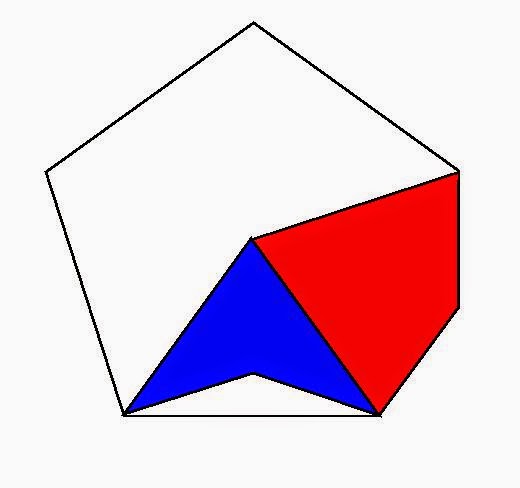
This tiling consists of two basic shapes, the kite and the dart, both derived by taking a canonical pentagon inscribed in a circle, splitting it into 5 triangles with common vertex the center of the circle, and then making a variation on one of these isosceles triangles: take the side of the triangle which corresponds to a chord of the circle and make an inwards bump to obtain the dart (blue figure below) and an outwards one to obtain the kite (red figure).
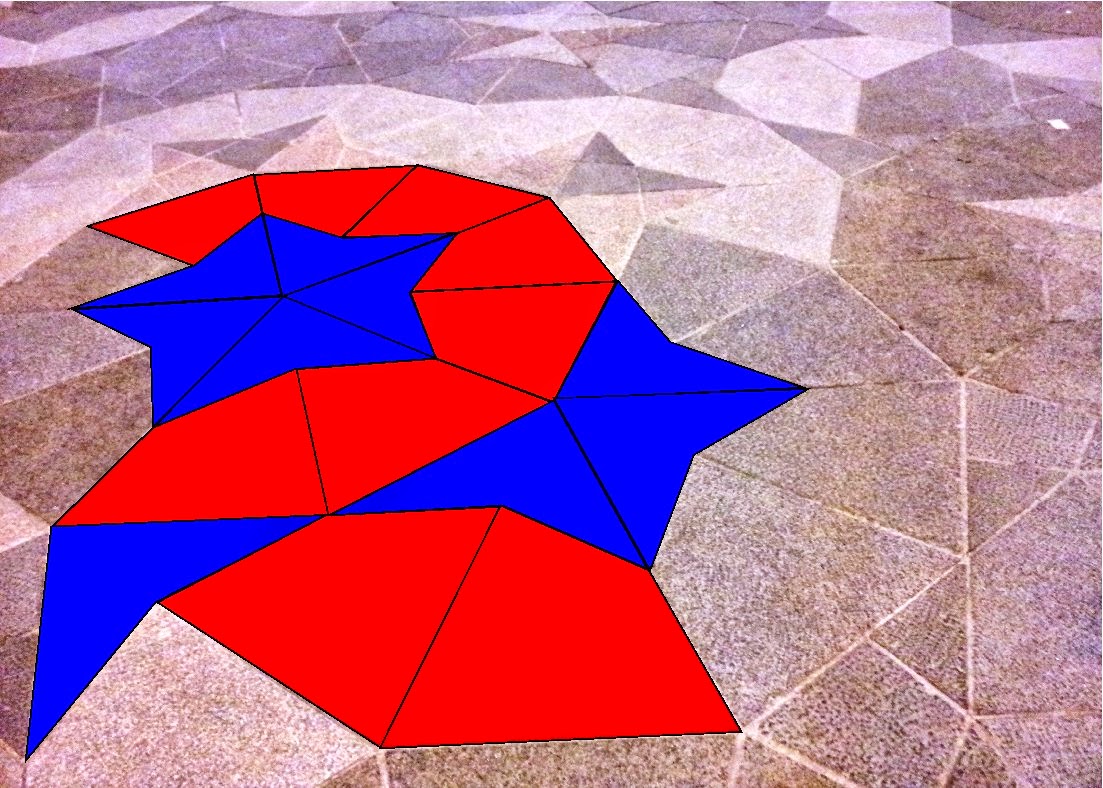
Then follow some rules on how to join copies of these pieces so as to completely cover the plane. The result is a non-periodic pattern: no finite portion of it can describe the whole tiling. In particular, the tiling has no translational symmetry and is self-similar. Here is another picture and below it my attempt to show you its basic shapes. Kites are red, darts are blue.
The interesting thing with this tiling is that it appears as if it will repeat itself after a while, but it won't (this is a theorem). Nevertheless it is not random because it is created from a set of specific rules.
The tiling was discovered first by Roger Penrose 40 years ago. It was known that one could produce non-periodic tilings with a finite number of shapes but Penrose managed to do this with only 2. In nature, there are materials (quasicrystals) exhibiting such behaviors. Since the Penrose tiling is based on the pentagon, the so-called golden ratio plays a fundamental role. Indeed, if we call A, B, C, D, E the vertices of a canonical pentagon (in the ordered traversed when going around in one direction) and let X be the point of the intersection of the chords AC and BE then, using similar triangles, we see that AX/AB = AB/AC. (The triangles ABX and ACB are similar, i.e., one is a scaled version of the other.) If we let AB=a and AX=b, then we see that AB=a and XC=a, so AC=AX+XC = b+a. The equality of the ratios above then becomes b/a = a/(a+b), so if we let φ be the ratio b/a, we have φ = 1/(1+φ) which means that φ2 + φ = 1. But (φ+(1/2))2 = φ2 + φ + (1/4) = 1 + (1/4) = 5/4, and so φ = (√5 -1)/2, a number known and used since times immemorial.
If you have java installed and enabled on your browser, you can play with trying to create variations of non-periodic tilings using the Penrose tiling applet. (Or see the PhD thesis of Craig Caplan.)
But the interesting thing is what a then young PhD postdoctoral physicist, Peter Lu, found out some 10 years ago in (the Islamic) Darb-i Imam shrine in Isfahan, Iran, dating from 1453. He observed that the patterns forming the wall decorations form a non-periodic tiling, just as the Penrose tiling. In fact, you can see the kites and darts in the picture below.
He then wrote a paper with (P Steinhardt) analyzing this.I think that, since then, non-periodic patterns have been discovered in other places in the Islamic world. And businesses have grown out of it.
The fascinating thing about this discovery is two-fold. First, its mathematical interest and the fact that non-periodic tilings had been discovered more than 500 years ago. Second, the fact that they had been discovered empirically. Which makes us wonder why on earth would those Muslim decorators be interested in creating something so complex. My reasoning is as follows. It is known that, in Islam, people are very restricted with what kind of things they are allowed to decorate their temples/mosques/shrines. Gods and the like are not allowed. Human forms are not allowed. Animals or plants are not allowed (exception: in Iran, but that is, I am being told, a remnant of the pre-Islamic religion). Any concrete objects are not allowed. This is why Muslims have very few things they can play with: abstract patterns, tilings, geometric figures. But, even within this restricted framework, humans' minds can be quite creative. Humans have an innate need to be free, to explore, to wonder, to create. When authority or religion impose restrictions and rules, humans will try as much as they can to break them, even unconsciously. It seems that this is a prime example of the innate need for freedom of expression.
This tiling consists of two basic shapes, the kite and the dart, both derived by taking a canonical pentagon inscribed in a circle, splitting it into 5 triangles with common vertex the center of the circle, and then making a variation on one of these isosceles triangles: take the side of the triangle which corresponds to a chord of the circle and make an inwards bump to obtain the dart (blue figure below) and an outwards one to obtain the kite (red figure).
Then follow some rules on how to join copies of these pieces so as to completely cover the plane. The result is a non-periodic pattern: no finite portion of it can describe the whole tiling. In particular, the tiling has no translational symmetry and is self-similar. Here is another picture and below it my attempt to show you its basic shapes. Kites are red, darts are blue.
The interesting thing with this tiling is that it appears as if it will repeat itself after a while, but it won't (this is a theorem). Nevertheless it is not random because it is created from a set of specific rules.
The tiling was discovered first by Roger Penrose 40 years ago. It was known that one could produce non-periodic tilings with a finite number of shapes but Penrose managed to do this with only 2. In nature, there are materials (quasicrystals) exhibiting such behaviors. Since the Penrose tiling is based on the pentagon, the so-called golden ratio plays a fundamental role. Indeed, if we call A, B, C, D, E the vertices of a canonical pentagon (in the ordered traversed when going around in one direction) and let X be the point of the intersection of the chords AC and BE then, using similar triangles, we see that AX/AB = AB/AC. (The triangles ABX and ACB are similar, i.e., one is a scaled version of the other.) If we let AB=a and AX=b, then we see that AB=a and XC=a, so AC=AX+XC = b+a. The equality of the ratios above then becomes b/a = a/(a+b), so if we let φ be the ratio b/a, we have φ = 1/(1+φ) which means that φ2 + φ = 1. But (φ+(1/2))2 = φ2 + φ + (1/4) = 1 + (1/4) = 5/4, and so φ = (√5 -1)/2, a number known and used since times immemorial.
If you have java installed and enabled on your browser, you can play with trying to create variations of non-periodic tilings using the Penrose tiling applet. (Or see the PhD thesis of Craig Caplan.)
But the interesting thing is what a then young PhD postdoctoral physicist, Peter Lu, found out some 10 years ago in (the Islamic) Darb-i Imam shrine in Isfahan, Iran, dating from 1453. He observed that the patterns forming the wall decorations form a non-periodic tiling, just as the Penrose tiling. In fact, you can see the kites and darts in the picture below.
He then wrote a paper with (P Steinhardt) analyzing this.I think that, since then, non-periodic patterns have been discovered in other places in the Islamic world. And businesses have grown out of it.
The fascinating thing about this discovery is two-fold. First, its mathematical interest and the fact that non-periodic tilings had been discovered more than 500 years ago. Second, the fact that they had been discovered empirically. Which makes us wonder why on earth would those Muslim decorators be interested in creating something so complex. My reasoning is as follows. It is known that, in Islam, people are very restricted with what kind of things they are allowed to decorate their temples/mosques/shrines. Gods and the like are not allowed. Human forms are not allowed. Animals or plants are not allowed (exception: in Iran, but that is, I am being told, a remnant of the pre-Islamic religion). Any concrete objects are not allowed. This is why Muslims have very few things they can play with: abstract patterns, tilings, geometric figures. But, even within this restricted framework, humans' minds can be quite creative. Humans have an innate need to be free, to explore, to wonder, to create. When authority or religion impose restrictions and rules, humans will try as much as they can to break them, even unconsciously. It seems that this is a prime example of the innate need for freedom of expression.









No comments:
Post a Comment